Euclid

• In the time of Euclid, it was considered improper mathematical etiquette to create constructions by simply lifting the compass and striking a mark somewhere else on the page. However, over time this perception changed and we now can lift the compass and place it somewhere else on the page. Of course, it is easy for modern students of geometry to consider this process archaic as we now have software on that can perform the same construction in a single step. Regardless of our opinions of the collapsible compass construction, Euclid chose to begin his elements with the most basic and elegant construction - how to copy a segment from one point in space onto another.

• A construction to copy the segment is presented in Euclid’s Proposition 2 of The Elements of Geometry. Mathematical historians question whether Euclid was truly a mathematician or simply a librarian. Because Euclid had access to many of the books available on the topic of Geometry, he was able to develop the first organized text devoted to the topic. How much of a contribution that Euclid made to The Elements would be pure speculation. However, it cannot be disputed that the organization of this text would take an understanding of Geometry that few students or even teachers of the subject will ever reach.

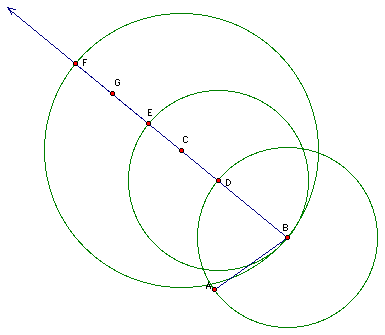
• The construction as presented in Euclid’s Elements is presented quite clearly at the web page of David E. Joyce, Professor of Mathematics and Computer Science at Clark University. Euclid’s construction requires the construction of an equilateral triangle and two circles in order to copy a segment to a random point.

• The construction I propose as an alternative to Euclid’s solution to the collapsible compass problem involves the construction of three circles and a midpoint. This construction I propose is the same for any case. However, the proofs for each case are different. In practice, this issue is really not a problem. If you are interested, you can download the proof of my Collapsible Compass Construction as a PDF file in worksheet format. To view the steps to construct this alternative pictured to the right, click here. You can also download a paper I wrote while in college on the topic of the Collapsible Compass Construction alternatives. I can provide assistance with any step of the construction by contacting me at teast@me.com. An algebraic proof of each case is available upon request.

Travis East